What is compound interest, and how can I calculate it?

At a time when the interest rates on many savings accounts are lower than they have been in the past, it can seem hard to make your money grow. But one thing that can help is taking advantage of compound interest. Here we look at how compound interest works and how you can calculate the effect it could have on your savings over time.
We all want our savings to grow, but in a low interest rate environment that can be a challenge.
One way to help is to take advantage of compound interest, something so powerful even Albert Einstein is said to have said a few words about it.
Compound interest can be good for your savings, but it can also be bad for your debts. That’s why compound interest and how it works is taught in Australian schools, to help young people be more financially literate.
Simple interest vs compound interest: what’s the difference?
Banks and other financial institutions use interest rates as a way to entice you to do business with them, such as depositing your money into their savings accounts or term deposits. As a general rule, the larger the interest rate a bank pays you, the more your savings will grow over time.
Banks calculate interest rates on each of their products in one of two different ways, called simple and compound interest.
→ Learn more: How is interest calculated on your home loan?
What is simple interest?
Some savings accounts pay what is called simple interest. That’s where you get a fixed interest payment over a set period of time. For example, say you invest $10,000 at 1% for 12 months, and at the end of the period you get $100 in interest.
Some term deposits can work this way, as well as several types of loans, provided you keep up with the repayments.
What is compound interest?
Compound interest is where you earn interest on top of any interest already earned on your savings. Most savings accounts and some term deposits in Australia work this way.
Rather than waiting until the end of a fixed period for interest to be paid, you can have it calculated and paid incrementally, say quarterly, monthly, weekly or even daily, depending on the particular account you choose. That way, your savings can grow even faster as the interest payments compound.
For example, if you have $10,000 invested at 1% per annum but choose to have that interest paid monthly, at the end of 12 months you would have earned $100.46 in interest instead of $100 as an annual payment.
That extra $0.46 might not sound like much, but the key to compound interest is to keep your savings earning interest on top of interest for some time.
After two years, you would have earned a total of $201.93 in interest, and after 10 years you would have earned $1051.25, excluding any potential fees and assuming your interest rate remained the same throughout that time. See the table below.
Compound interest over time on a $10,000 investment
| Years | Value | Interest |
|---|---|---|
| 1 | $10,100.46 | $100.46 |
| 2 | $10,201.93 | $201.93 |
| 5 | $10,512.49 | $512.49 |
| 10 | $11,051.25 | $1,051.25 |
| 25 | $12,838.92 | $2,838.92 |
Source: www.canstar.com.au. Assumes a starting balance of $10,000, an annual interest rate of 1% and that the interest is paid into the account at the end of each month.
So after 25 years, that $10,000 in this scenario would have earned $2,838.92 in interest and you would have $12,838.92 in your savings account, largely thanks to compound interest.
Obviously interest rates may change over that 25-year period so you could end up with more, or less, money than that. But the hope is that your savings will grow and keep pace with inflation in the long run.
Dr Campbell Heggen, a senior lecturer in financial planning at Deakin University, said it’s a good idea to look for interest rates that are equal or greater than inflation, if possible.
“While compounding can have the effect of increasing the real rate of return on your savings, an account offering a mere fraction of a per cent per annum will never keep pace with inflation – regardless of how often your returns are compounded,” he told Canstar.
→ Learn more: Canstar’s Term Deposit Calculator
How to boost your compound interest
Even if low interest rates mean your savings might only grow close to, or in line with inflation, there are other steps you can take if you want your savings to grow further.
One way to do that is to add to your savings over time, with regular payments if you can afford them.
“The more you deposit, the higher the value interest will be calculated against,” said Dr Heggen.
“A diligent savings plan is likely going to reap greater long-term benefits than relying on interest alone to grow your savings.”
If we take the initial $10,000 investment example and can add an extra $100 a month, then after 12 months your savings could have grown to $11,305.97, including an extra $105.97 in interest. The table below shows how that value increases over time, again excluding any fees and assuming the 1% interest rate remains constant.
Compound interest over time with extra $100 monthly deposits
| Years | Value | Interest |
|---|---|---|
| 1 | $11,305.97 | $105.97 |
| 2 | $12,625.07 | $225.07 |
| 5 | $16,662.40 | $662.40 |
| 10 | $23,666.24 | $1,666.24 |
| 25 | $46,905.93 | $6,905.93 |
Source: www.canstar.com.au. Assumes a starting balance of $10,000, an annual interest rate of 1% and that the extra deposits and interest are paid into the account at the end of each month.
If you could afford monthly payments of up to $1,000, we can see how your savings could grow even more.
Compound interest over time with extra $1,000 monthly deposits at 1% interest
| Years | Value | Interest |
|---|---|---|
| 1 | $22,155.61 | $155.61 |
| 2 | $34,433.34 | $433.34 |
| 5 | $72,011.54 | $2,011.54 |
| 10 | $137,201.12 | $7,201.12 |
| 25 | $353,509.01 | $43,509.01 |
Source: www.canstar.com.au. Assumes a starting balance of $10,000, an annual interest rate of 1% and that the extra deposits and interest are paid into the account at the end of each month.
Each of these scenarios is based on an annual interest rate of 1%, which is low by historical standards. If interest rates were to rise in future, then so would the effect of compound interest, but remember to look for ways to boost your savings so they keep pace with, and hopefully ahead, of inflation.
Some institutions may offer extra bonus interest rates if you make regular deposits into your savings, but take care to check the terms and conditions to see if these accounts suit your needs.
→ Learn more: The highest-interest savings accounts on Canstar’s database
The downside of compound interest
Now you can see how compound interest can help you grow your savings, but it’s also important to understand it can also have an impact on any money you owe.
“This is particularly true for personal debt such as credit cards, car loans, and the various payment services which allow you to buy now and pay later,” said Dr Heggen.
But knowing this downside can help you reduce your debts. Similar to the way you can boost the effect of compound interest on your savings, you can reduce its impact on your debts by making extra payments above any minimum repayment required.
That way you can pay off your credit card or loan much faster. The Federal Government’s Moneysmart credit card calculator can help you work out the potential benefits of making extra payments.
“Generally speaking, paying off expensive short-term debt first usually has a much larger influence on your overall net worth than putting money aside in a low-rate savings account,” Dr Heggen added.
Can I calculate compound interest myself?
It is possible to calculate the effects of compound interest yourself, using a mathematical formula.
Compound interest has been part of the Australian school curriculum for the past 10 years and Allan Dougan, CEO of the Australian Association of Mathematics Teachers, said it is taught from a financial point of view.
“Compound interest is one of those skills that’s really important to understand and how to calculate it,” he told Canstar.
“It’s one of those times where there is mathematics that’s really relevant to young people. We need young people to be financially literate and have the financial capabilities.
“We know that young people will enter a world where there is debt and savings. To understand what’s going to save them the most savings or cost them the most debt is important.”
Mr Dougan said there are a number of online calculators available to help you calculate compound interest, though he cautions that some can produce different results. There is one on the Moneysmart website.
Or you can calculate the amount of compound interest you’d expect to earn using a little mathematics, especially if you were taught how at school. If you know your way around a calculator or a spreadsheet that can help too.
How to calculate compound interest using a formula
Let’s keep our example of investing $10,000 and an annual interest rate of 1%.
We can use this formula to calculate the expected future value of your investment from any compound interest.
Future value = A × ( ( 1 + ( R ÷ P ) ) ^ ( Y × P ) )
A is the amount of your initial investment, R is the interest rate per annum, Y is the number of years you wish to invest and P is the period of any interest to be paid, or in other words how often it is paid each year. So if you want your interest paid annually, P=1, if you want it quarterly P=4 and if you want it monthly P=12.
The × symbol means multiply and the ÷ symbol means divide. Alternatively, you can type * for multiplication and / for division, which may be easier on some keyboards.
The ^ symbol means something is raised to the power, or to the order, or in other words multiplied by itself a certain number of times. For instance, two to the power of three would be 2 × 2 × 2 = 2 ^ 3 = 8. Some calculators allow you to do such calculations. Don’t worry if yours doesn’t. Look for a button with the symbol xy (or yx on some calculators). If you’re using a computer or phone which doesn’t have this option, you can use the ^ symbol.

What is important here is to remember the basic rule in mathematics about the order in which calculations in any formula should be done. One way to remember the order is the mnemonic BODMAS: you tackle things inside Brackets first, then Orders, Division, Multiplication, Addition and Subtraction.
The easiest way to do such a calculation is to break down the formula into parts.
Say we want to invest our $10,000 over five years, with interest paid monthly.
A = $10,000
R = 1% (which we need to convert to a decimal, see later)
P = 12
Y = 5
Let’s work on the things inside brackets first and calculate ( 1 + ( R ÷ P ) ).
Percentages are just fractions of 100, so 1% is actually 1/100 = 0.01. If it was 2% then that would be 2/100 = 0.02, 5% would be 0.05 and so on. We also need to remember from BODMAS that we do the division first and then the addition.
So now ( 1 + ( R ÷ P ) ) = 1 + 0.01 ÷ 12 = 1 + 0.0008333… (the 3 continues but we can stop it after three repeats, or store the figure in your calculator’s memory) = 1.0008333
Next, we tackle the other brackets ( Y * P ) = 5 × 12 = 60, meaning there will be 60 monthly interest payments over the five years.
Now we’ve done the brackets, we need to do the Order calculation, that’s the ^ symbol.
So ( 1 + ( R ÷ P ) ) ^ ( Y × P ) = 1.0008333 ^ 60 = 1.051247…
Finally, we multiply this by our initial investment to work out the ‘future value’ – the total amount that would be in our account after the five years in this scenario.
Future value = A × 1.051247 = $10,512.47
Which is pretty close to the figure in our original table (the slight difference is due to rounding errors where we cut off part of the number in our calculation).
This formula works for just an initial amount that’s invested over time. If you want to make extra regular payments, then you probably need to use a spreadsheet, or an online calculator like Moneysmart’s, which allows you to factor in these additional deposits.
How to use a spreadsheet to calculate compound interest
Most spreadsheet software has a function known as FV, for Future Value. Check the help section of your software to find out how it works for you, as they can vary in the way the data is entered into the function.
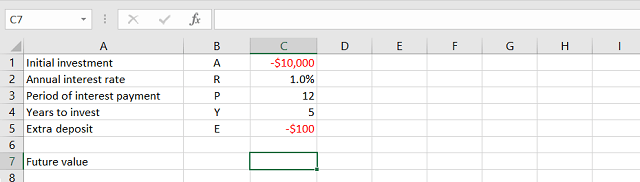
Let’s stick with our previous example, but add an E for the extra amount you intend to deposit on a regular basis (we’ll assume $100 per month in this example).
A = $10,000
R = 1%
P = 12
Y = 5
E = $100
The example here is using Microsoft’s Excel which is available for most computers, often as part of the Microsoft Office suite.
First we enter all the data into cells in the spreadsheet.
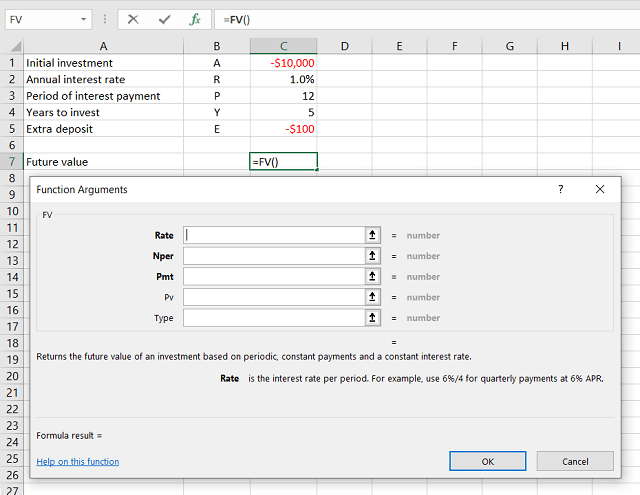
It is a quirk of spreadsheet software that the initial amount and the extra deposit to be made each period are entered as negative numbers. When done, click on the cell where you want the function value to appear, C7 in our case
A good way to locate the FV function is to use the fx option, located just above column B in the image above. If you click on this, it should bring up a box where you can enter the relevant parts of your data.
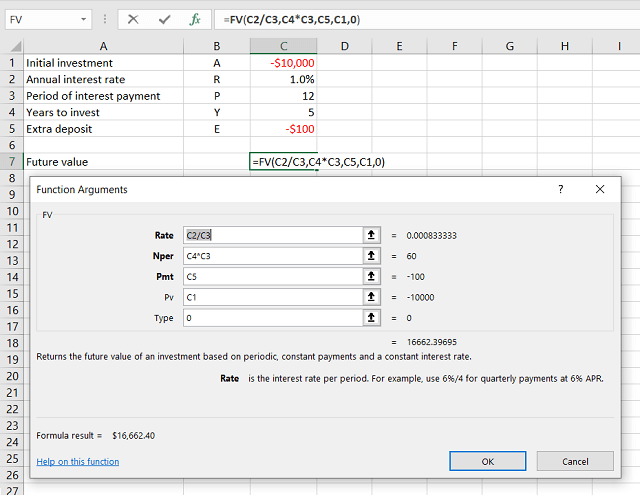
With Rate we need to divide the annual interest rate by the period of payment, which in this example is monthly, so Rate = cell C2 divided by cell C3. So enter C2/C3 in the Rate box.
We need to do something similar for Nper which is the number of payments to be paid over the length of the investment, so Nper is cell C4 multiplied by C3. Enter C4*C3 in the Nper box.
Pmt is the extra deposit amount you wish to invest so enter cell C5 in the Pmt box, and Pv is the original amount of your investment so enter cell C1 in the Pv box.
For the last argument, Type, you can either leave it blank or enter a 0 (zero) to indicate you want the payments and interest added at the end of each month.
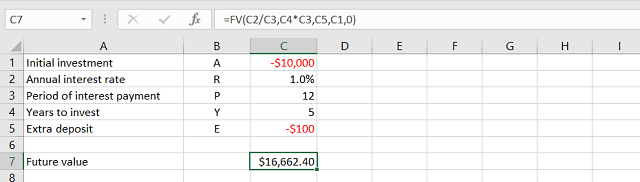
Click the OK button and the function will work out the Future Value of your investment after five years, with interest paid every month and extra payments of $100.
If you want to change the value of your extra payment then change the number in cell C5 (if cell C5 is 0 (zero), this is the same as the compound interest calculator without extra payments we did earlier).
You can also change the number of years you want the investment to run by changing the number in cell C4, or if you want the interest and extra deposit paid quarterly instead of monthly then change the number in cell C3 to a 4.
Now that you’re armed with the know-how to create your own compound interest calculator , you can use it to help you work out the best way for you to grow your savings based on your circumstances.
What did Albert Einstein say about compound interest?
The Nobel Prize winning theoretical physicist Albert Einstein was certainly a genius, and probably understood the importance of compound interest better than many.

Search the web and you will find many sites attributing various quotes on compound interest to Einstein, such as this example from Goodreads:
“Compound interest is the eighth wonder of the world. He who understands it, earns it … he who doesn’t … pays it.”
But did Einstein really say that?
Probably not, according to the Quote Investigator website, which says the “eighth wonder” phrase appears in the ‘Probably Not By Einstein’ section in the book The Ultimate Quotable Einstein, published by Princeton University Press.
Still, now you have the knowledge to benefit from the second part of the quote Einstein probably didn’t say either.
Cover image source: Zephyr_p/Shutterstock.com
This article was reviewed by our Digital Editor Amanda Horswill and Sub Editor Tom Letts before it was updated, as part of our fact-checking process.

Michael is an award-winning journalist with more than three decades of experience. As a senior finance journalist at Canstar, Michael wrote more than 100 articles covering superannuation, savings, wealth, life insurance and home loans. His work's been referenced by a number of other finance publications, including Yahoo Finance and The Motley Fool.
Michael's worked as a reporter and producer for the BBC and ABC, including for Australian Story. He's also worked as a feature writer for The Courier-Mail and as a science and technology editor and commissioning editor at The Conversation.
Michael's professional awards include a Queensland Media Award and a highly commended in the Walkleys. In 2021 he was part of a team that was a finalist in the Australian Museum Eureka Prize for Science Journalism. He holds a Bachelor of Science in mathematics and applied physics (Manchester Metropolitan University) and a Masters of Science in pure mathematics (Liverpool University).
You can connect with Michael on LinkedIn.
- Simple interest vs compound interest: what’s the difference?
- How to boost your compound interest
- The downside of compound interest
- Can I calculate compound interest myself?
- How to calculate compound interest using a formula
- How to use a spreadsheet to calculate compound interest
- What did Albert Einstein say about compound interest?
Try our Savings Accounts comparison tool to instantly compare Canstar expert rated options.